Please refer to Chapter 2 Polynomials MCQ Class 9 Mathematics with answers below. These multiple-choice questions have been prepared based on the latest NCERT book for Class 9 Mathematics. Students should refer to MCQ Questions for Class 9 Mathematics with Answers to score more marks in Grade 9 Mathematics exams. Students should read the chapter Polynomials and then attempt the following objective questions.

MCQ Questions Class 9 Mathematics Chapter 2 Polynomials

The Polynomials MCQ Class 9 Mathematics provided below covers all important topics given in this chapter. These MCQs will help you to properly prepare for exams.

Question. One of the zeroes of the polynomial 2x² + 7x – 4 is
(a) 2
(b) 1/2
(c) − 1/2
(d) −2

Question. √2 is a polynomial of degree
(a) 2
(b) 0
(c) 1
(d) 1/2

Question. Degree of the polynomial 4x⁴+ 0x³+ 0x⁵+ 5x + 7 is
(a) 4
(b) 5
(c) 3
(d) 7

Question. The factorization of 4x²+ 8x + 3 is
(a) (x + 1) (x + 3)
(b) (2x + 1) (2x + 3)
(c) (2x + 2) (2x + 5)
(d) (2x –1) (2x –3)

Question. Zero of the zero polynomial is
(a) 0
(b) 1
(c) any real number
(d) not defined

Question. If x/y + y/x =−1 ( x, y ≠ 0) , the value of x³– y³ is :
(a) 1
(b) −1
(c) 0
(d) 1/2

Question. The value of 2492 – 2482 is :
(a) 12
(b) 477
(c) 487
(d) 497

Question. Which one of the following is a polynomial ?
(a) x²/2 − 2/x²
(b) √2x − 1
(c) x² + 3x³/2/√x
(d) x − 1/ x + 1

Question. If a + b + c = 0, then a³+ b³+ c³ is equal to
(a) 0
(b) abc
(c) 3abc
(d) 2abc

Question. If p (x) = x² − 2√2x + 1, then p(2√2) is equal to
(a) 0
(b) 1
(c) 4√2
(d) 8√2 + 1

Question. The coefficient of x in the expansion of (x + 3)³ is :
(a) 1
(b) 9
(c) 18
(d) 27

Question. The coefficient of x in the product (x – 1)(1 – 2x) is :
(a) 2
(b) – 1
(c) – 2
(d) 1

Question. The degree of the polynomial 2 – y² – y³ + 2y⁷ is :
(a) 2
(b) 7
(c) 0
(d) 3

Question. The degree of the polynomial p(x) + (x – 7)³ – x³ is :
(a) 3
(b) 2
(c) 1
(d) 0

Question. A polynomial p(x) of degree n is such that p(a) = 0 and p(–b) = 0. Which of the following is the factored form of the polynomial?
(a) (x – a)(x + b) g(x); where g(x) is a polynomial of degree n – 2.
(b) (x – a)(x + b) g(x); where g(x) is a polynomial of degree n
(c) (x + a)(x + b) g(x); where g(x) is a polynomial of degree n – 2.
(d) (x + a)(x + b) g(x); where g(x) is a polynomial of degree n. 

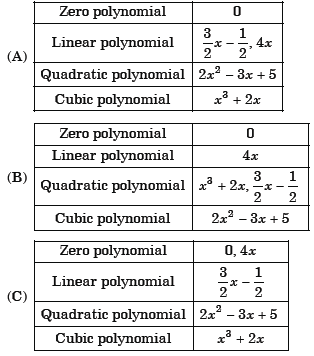
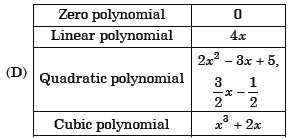
 Question. Consider the polynomials shown :
x³ + 2x, 4x, 2x² – 3x + 5 =0 , 3/2x – 1/2
Which of the following tables correctly classifies the given polynomials as zero, linear, quadratic and
cubic polynomials?

Question. Which of the following is a quadratic polynomial in one variable ?
(a) √2x³ + 5
(b) 2x² + 2x^-2
(c) x²
(d) 2x² + y²

Question. Which of the following is a trinomial in x ?
(a) x³+ 1
(b) x³+ x² + x
(c) x√x + √x + 1
(d) x³+ 2x

Question. The polynomial (x – a) is a factor of the polynomial x⁴ – 2x² + kx + k, where k is a constant. Which of these is the correct relation between a and k ?
(a) k = a²(2 – a²)/1 + a
(b) k = a²(2 + a²)/1 + a
(c) k = a²(2 + a²)/1 – a
(d) k = a²(2 – a²)/1 – a

Question. Which of these is a factor of the polynomial p(x) = x³ + 4x + 5 ?
(a) (x – 1)
(b) (x + 1)
(c) (x – 2)
(d) (x + 2) 

Question. If p(x) = 2 + x/2 + x² – x³/3 , then p(– 1) is :
(a) 15/6
(b) 17/6
(c) 1/6
(d) 13/6 

Question. Given that m + 2, where m is a positive integer, is a zero of the polynomial q(x) = x² – mx – 6. Which of these is the value of m ?
(a) 1
(b) 2
(c) 3
(d) 4 

Question. Zero of the polynomial p(x), where p(x) = ax, a≠0 is :
(a) 1
(b) a
(c) 0
(d) 1/a 

Question. (1 + 3x)³ is an example of :
(a) monomial
(b) binomial
(c) trinomial
(d) none of these

Question. Which of the following is a polynomial?
(a) x²/3 – 5/x²
(b) 2x+1/3x+4
(c) √5x + 7
(d) x³ + 3x^5/2/√x

Question. Match the columns 

(a) 1 (c); 2 (e); 3 (d); 4 (a); 5 (b)
(b) 1 (d); 2 (e); 3 (a); 4 (c); 5 (b)
(c) 1 (e); 2 (d); 3 (b); 4 (c); 5 (a)
(d) 1 (b); 2 (e); 3 (a); 4 (c); 5 (d)

Case based MCQs

Read the following text and answer the following questions on the basis of the same:
Beti Bachao, Beti Padhao (BBBP) is a personal campaign of the Government of India that aims to generate awareness and improve the efficiency of welfare services intended for girls.

In a school, a group of (x + y) teachers, (x² + y²⁾ girls and (x³ + y³) boys organised a campaign on Beti Bachao, Beti Padhao.

Question. (x + y)³ =
(a) x³– y³ – 3xy(x + y)
(b) x³+ y³ + 3xy(x + y)
(c) x³+ y³ – 3xy(x – y)
(d) x³– y³ + 3xy(x – y)

Question. If in the group, there are 10 teachers and 58 girls, then what is the number of boys?
(a) 300
(b) 360
(c) 350
(d) 370

Question. Which mathematical concept is used here?
(a) Linear equations
(b) Triangles
(c) Polynomials
(d) Area

Question. Using part (d), find (x²– y²) if x – y = 23.
(a) 200
(b) 330
(c) 120
(d) 230

Question. Which is the correct identity?
(a) (a + b)² = a² + b² – 2ab
(b) (a + b)² = a²+ b² + 2ab
(c) (a + b)² = a²– b² – 2ab
(d) All are correct.

Read the following passage and answer the questions.
Smriti went to a orphanage and gave a cuboidal gift whose volume was given by a polynomial 4x³ + x² – 11x + 2r. 

Question. What could be the breadth and height of the cuboid?
(a) (x + 2) and (x – 1)
(b) (x – 2) and (x + 1)
(c) (x + 2) and (x + 1)
(d) (x – 2) and (x – 1) 

Question. If length of the cuboid is (4x – 3), then value of r is
(a) 11
(b) 4
(c) 3
(d) 2 

Question. Cost of the gift given by Smriti at the rate of 5 per cubic units will be
(a) 180
(b) 90
(c) 900
(d) 450 

Question. Degree of the polynomial is
(a) 4
(b) 3
(c) 2
(d) 1 

Question. If x = 3, then dimensions of the cuboid will be
(a) 9, 1 and 4
(b) 9, 5 and 2
(c) 9, 5 and 4
(d) 9, 1 and 2