Triangles MCQ Class 10 Mathematics
Please refer to Chapter 6 Triangles MCQ Class 10 Mathematics with answers below. These multiple-choice questions have been prepared based on the latest NCERT book for Class 10 Mathematics. Students should refer to MCQ Questions for Class 10 Mathematics with Answers to score more marks in Grade 10 Mathematics exams. Students should read the chapter Triangles and then attempt the following objective questions.
MCQ Questions Class 10 Mathematics Chapter 6 Triangles
The Triangles MCQ Class 10 Mathematics provided below covers all important topics given in this chapter. These MCQs will help you to properly prepare for exams.
Question. If in two triangles ABC and PQR, AB/QR= BC/PR= CA/PQ , then
(a) ΔPQR ~ ΔCAB
(b) ΔPQR ~ ΔABC
(c) ΔCBA ~ ΔPQR
(d) ΔBCA ~ ΔPQR
Answer
A
Question. If ΔPRQ ~ ΔXYZ, then
(a) PR/XZ= RQ/YZ
(b) PQ/XY= PR/XZ
(c) PQ/XZ= QR /YZ
(d) QR/XZ= PR/XY
Answer
C
Question. A square and a rhombus are always
(a) similar
(b) congruent
(c) similar but not congruent
(d) neither similar nor congruent
Answer
D
Question. In triangles ABC and DEF, ∠B = ∠E, ∠F = ∠C and AB = 3DE. Then, the two triangles are
(a) congruent but not similar
(b) similar but not congruent
(c) neither congruent nor similar
(d) congruent as well as similar
Answer
B
Question. Vatsal claims that congruent figures are similar as well. Vinayak claims that similar figures are congruent as well. Who is/are correct?
(a) Only Vatsal
(b) Only Vinayak
(c) Both Vatsal and Vinayak
(d) Neither Vatsal nor Vinayak
Answer
A
Question. It is given that ΔABC ~ ΔDFE, ∠A = 30°, ∠C = 50°, ΔAB = 5 cm, AC = 8 cm and DF = 7.5 cm. Then, which of the following is true?
(a) DE = 12 cm, ∠F = 50°
(b) DE = 12 cm, ∠F = 100°
(c) EF = 12 cm, ∠D = 100°
(d) EF = 12 cm, ∠D = 30°
Answer
B
Question. Two circles are always
(a) congruent
(b) neither similar nor congruent
(c) similar but may not be congruent
(d) none of these
Answer
C
Question. In figure, two line segments AC and BD intersect each other at the point P such that PA= 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to
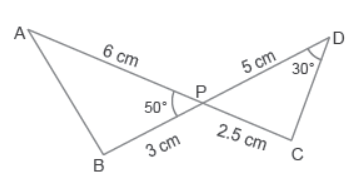
(a) 50°
(b) 30°
(c) 60°
(d) 100°
Answer
D
Question. If ΔABC ~ ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
(a) BC. EF = AC. FD
(b) AB. EF = AC. DE
(c) BC. DE = AB. EF
(d) BC. DE = AB. FD
Answer
C
Question. In ΔABC and DRPQ, AB = 4.5 cm, BC = 5 cm, CA = 6 2cm, PR = 12 2 cm, PQ = 10 cm, QR = 9 cm. If ∠A = 75° and ∠B = 55°, then ∠P is equal to
(a) 75°
(b) 55°
(c) 50°
(d) 130°
Answer
C
Question. If ΔABC ~ ΔEDF and DABC is not similar to ΔDEF, then which of the following is not true?
(a) BC . EF = AC . FD
(b) AB . EF = AC . DE
(c) BC . DE = AB . EF
(d) BC . DE = AB . FD
Answer
C
Question. If ΔPQR ~ ΔXYZ and PQ/XY= 5/2 , then (ar ΔXYZ ) (ar ΔPQR ) is equal to
(a) 4/25
(b) 2/5
(c) 25/4
(d) 5/2
Answer
A
Question. In the given figure, QR || AB, RP || BD, CQ = x + 2, QA = x, CP = 5x + 4, PD = 3x. The value of x is _____________.
(a) 1
(b) 6
(c) 3
(d) 9
Answer
A
Question. It is given that ar(ΔABC) = 81 square units and ar(ΔDEF) = 64 square units. If DABC ~ DDEF, then
(a) AB/DE= 81/64
(b) AB2/DE2= 9 /2
(c) AB/DE= 9/8
(d) AB = 81 units, DE = 64 units
Answer
C
Question. In the given figure, AD is the bisector of ∠BAC. If AB= 10 cm, AC = 14cm and BC = 6 cm, find the length of DC.
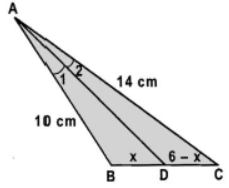
(a) 2 cm
(b) 3.5 cm
(c) 2.5 cm
(d) 4 cm
Answer
B
Question. Lf ΔABC~ ΔDEF , BC=3cm,EF = 4cm and area of ΔABC = 54cm2 , find the area of ΔDEF.
(a) 96 m2
(b) 16 cm2
(c) 96 cm2
(d) 69 cm2
Answer
C
Question. In ∠ABC , DE || BC and AD/DB = 3/5 . What is the length of AE if AC = 4.8cm?
(a) 1.4cm
(b) 1.8cm
(c) 2.2 cm
(d) 3.6 cm
Answer
B
Question. In the given figure, AO/OC = BO/OD = 1/2 and AB = 5 cm. Find the value of DC.

(a) 5 cm
(b) 15cm
(c) 10 cm
(d) 8 cm
Answer
C
Question. In the given figure, AABC is right angled at A. DEFG is a square, BD = 12 cm and EC = 27 cm. What is the length of the side of square?

(a) 27 cm
(b) 19 cm
(c) 12 cm
(d) 18 cm
Answer
D
Question. In the given figure, ΔABCis right angled at A.
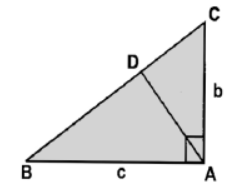
What is the length of AD in terms of ‘b’ and ‘c’?
(a) AC/√b2 + c2
(b) bc/√b2 + c2
(c) bc/√b2 – c2
(d) bc/√c2 – b2
Answer
B
Question. A paper is cut in the following shape.
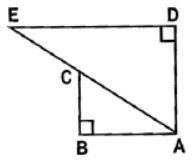
Which of the following is the correct statement?
(a) AE2 = AD2 + ED2
(b) AC2 = AD2 + DE2
(c) AC2 = AB2 + AD2
(d) AE2 = AB2 + BC2
Answer
A
Question. ΔABC is right-angled at C. If p is the length of perpendicular from C to AB and AB = c, BC = a and CA = b, which of the following is true?
(a) pc = ab
(b) pb = ab
(c) pc = bc
(d) pb = ac
Answer
A
Question. In the figure, DE| | BC . Find AE.
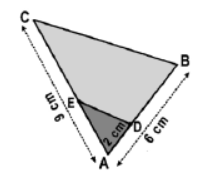
(a) 6 cm
(b) 4 cm
(c) 5 cm
(d) 3 cm
Answer
D
Question. In similar triangles ΔABC and ΔFDE.DE = 4 cm, BC = 8 cm and area of ΔFDE = 25 cm2 . What is the area of ΔABC?
(a) 144 cm2
(b) 121 cm2
(c) 100 cm2
(d) 81 cm2
Answer
C
Question. In the given figure, quad. ABCD~ quad PQRS. Find the value of ‘z’.

(a) 5 ,4/6
(b) 45/4
(c) 5 ,5/6
(d) 35/3
Answer
C
Question. In the given figure, two line segments AC and BD intersect each other at point P, such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30° . D Find ∠PBA .
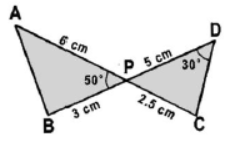
(a) 70°
(b) 80°
(c) 90°
(d) 100°
Answer
D
Question. If ΔABC and ΔPQR are similar and BC/QR = 1/3 , find ar (ΔPQR)/ar (ΔBCA).
(a) 9
(b) 3
(c) 1/3
(d) 1/9
Answer
A
Question. Aman drives 13 km in the north west direction, turns left and drives 5 km. How far is he from the starting point?
(a) 10km
(b) 18km
(c) 12 km
(d) 11 km
Answer
C
Question. In the figure, ∠ABC = ∠DAC = 90° , BC = 3 cm, AD = 2 cm and AB = 1 cm. What is the measure of AC + CD?

(a) √2( √5 + √7)cm
(b) √5( √2 + √7)cm
(c) √2( √7 + √5)cm
(d) √7( √2 + √2)cm
Answer
A
Question. ΔABCis right angled at C and AC = √3BC . What is the value of ∠ABC?
(a) 30°
(b) 90°
(c) 60°
(d) 45°
Answer
C
Question. ΔABC and ΔBDE are two equilateral triangles such that D is the mid-point of BC. Ratio of the area of triangles ABC and BDE is
(a) 2 : 1
(b) 1 : 2
(c) 1 : 4
(d) 4 : 1
Answer
D
Question. In triangle ABC, if AB = 6 √3 cm, AC = 12 cm and BC = 6 cm, then ∠B is
(a) 120°
(b) 60°
(c) 90°
(d) 45°
Answer
C
Question. If ΔABC ∼ ΔDEF such that AB = 1.2 cm and DE = 1.4 cm, the ratio of the areas of ΔABC and ΔDEF is
(a) 49 : 36
(b) 6 : 7
(c) 7 : 6
(d) 36 : 49
Answer
D
Question. In ΔABC, DE ll BC in given figure, then BD is equal to
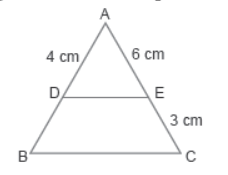
(a) 2 cm
(b) 8 cm
(c) 3 cm
(d) 9 cm
Answer
A
Question. Consider the statements below.
(i) All circles are similar.
(ii) All squares are similar.
(iii) All right triangles are congruent.
(iv) All equilateral triangles are congruent.
Which statement is/are correct?
(a) (i) and (ii)
(b) (ii) and (iv)
(c) (i) and (iii)
(d) (iii) and (iv)
Answer
A
Question. If Manish goes 3 km towards East and then 4 km towards North. His distance from starting point is
(a) 3 km
(b) 4 km
(c) 5 km
(d) 2 km
Answer
C
Question. In a triangle ABC if AB = 13 cm, BC = 12 cm and AC = 5 cm, then the triangle is right angled at
(a) A
(b) B
(c) C
(d) can’t say
Answer
C
Question. Observe the two triangles shown below.

Which statement is correct?
(a) Triangles are similar by SSA
(b) Triangles are similar by SAS
(c) Triangles are not similar as sides are not in proportion
(d) No valid conclusion about similarity of triangles can be made as angle measures are not
Answer
B
Question. If ΔABC ~ ΔPQR and (ar ΔABC )/(ar ΔPQR )= 9/4, also AB = 4 cm, then PQ is equal to
(a) 3 8 units
(b) 2 8 units
(c) 2 3 units
(d) 8 units
Answer
A
Question. If ΔABC ~ ΔQRP, (ar ΔABC)/ (ar ΔPQR)= 9/4 , AB = 18 cm and BC = 15 cm, then PR is equal to
(a) 10 cm
(b) 12 cm
(c) 20/3 cm
(d) 8 cm
Answer
A
Question. The length of each side of a rhombus whose diagonals are of lengths 10 cm and 24 cm is
(a) 25 cm
(b) 13 cm
(c) 26 cm
(d) 34 cm
Answer
B
Question. In ΔLMN and ΔPQR, ∠L = ∠P, ∠N = ∠R and MN = 2QR. Then the two triangles are
(a) Congruent but not similar
(b) Similar but not congruent
(c) neither congruent nor similar
(d) Congruent as well as similar
Answer
B
Question. It is given that ΔABC ~ ΔPQR, with BC/QR= 1/3 . Then, (ar ΔPRQ )/ (ar ΔBCA) is equal to
(a) 9
(b) 3
(c) 1/3
(d) 1/9
Answer
A
Question. If S is a point on side PQ of a ΔPQR such that PS = QS = RS, then
(a) PR . QR = RS2
(b) QS2 + RS2 = QR2
(c) PR2 + QR2 = PQ2
(d) PS2 + RS2 = PR2
Answer
C
Question. If in two triangles DEF and PQR, ∠D = ∠Q and ∠R = ∠E, then which of the following is not true?
(a) EF/PR= DF/PQ
(b) DE/PQ= EF/RP
(c) DE/QR= DF/PQ
(d) EF/RP= DE/QR
Answer
B
Question. Given that ΔABC~ΔDEF. If DE = 2AB and BC = 3 cm then, EF equals
(a) 12 cm
(b) 2 cm
(c) 1.5 cm
(d) 6 cm
Answer
D
Question. The straight line distance between A and B is (See figure)
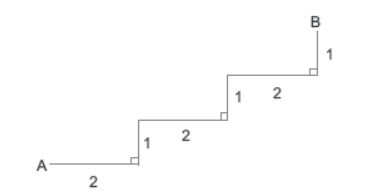
(a) 5 3 units
(b) 5 units
(c) 3 5 units
(d) 5 2 units
Answer
C
Question. Which set of lengths forms a right triangle?
(a) 5 cm, 12 cm, 16 cm
(b) 7 cm, 24 cm, 25 cm
(c) 3 cm, 3 cm, 4 cm
(d) 6 cm, 7 cm, 9 cm
Answer
B
Question. In a ΔABC,+A = 25°,+B = 35° and AB = 16 units. In ΔPQR,+P = 35°, +Q = 120° and PR = 4 units. Which of the following is true?
(a) ar (ΔABC) = 2ar (ΔPQR)
(b) ar (ΔABC) = 4ar (ΔPQR)
(c) ar (ΔABC) = 8ar (ΔPQR)
(d) ar (ΔABC) = 16ar (ΔPQR)
Answer
D
Question. The altitude of an equilateral triangle, having the length of its side as 12 cm, is
(a) 6 √2 cm
(b) 6 cm
(c) 8.5 cm
(d) 6 √3 cm
Answer
D
Question. In the adjoining figure, D, E and F are the mid-points of the side BC, AC and AB respectively of ΔABC then ar(ΔDEF) : ar(ΔABC) is
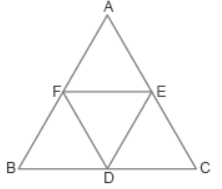
(a) 2 1
(b) 4 1
(c) 9 1
(d) None of these
Answer
B
Question. All the equilateral triangles are
(a) similar
(b) congruent
(c) both (a) and
(b) (d) none
Answer
A
Question. A ΔPQR is similar to another triangle ABC such that ar(ΔPQR) = 4ar(ΔABC) . The ratio of their perimeter is given as
(a) 2 : 1
(b) 1 : 2
(c) 4 : 1
(d) none of these
Answer
A
Question. If the three sides of a triangle are a, √3 a, √2 a then the measure of the angle opposite to the longest side is
(a) 60°
(b) 90°
(c) 45°
(d) 30°
Answer
B
Question. If in ΔABC and ΔDEF, AB/BC= DE/FD , then both will be similar when
(a) ∠B = ∠E
(b) ∠A = ∠D
(c) ∠B = ∠D
(d) ∠A = ∠F
Answer
C
Question. It is given that ΔABC ~ ΔDFE, ∠A = 30°, ∠C = 50°, AB = 5 cm, AC = 8 cm and DF = 7.5 cm. Then, which of the following is true.
(a) DE = 12 cm, ∠F = 50°
(b) DE = 12 cm, ∠F = 100°
(c) EF = 12 cm, ∠D = 100°
(d) EF = 12 cm, ∠D = 30°
Answer
B
Question. In ΔABC, AB = 6 √7 cm, BC = 24 cm and CA = 18 cm. Then angle A is
(a) an acute angle
(b) an obtuse angle
(c) a right angle
(d) can’t say
Answer
C
Question. QA and PB are perpendicular on AB, if AO = 10 cm, BO = 6 cm and PB = 9 cm, then measure of AQ (see figure) is
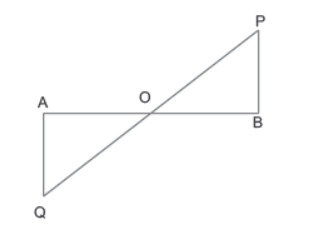
(a) 15 cm
(b) 25 cm
(c) 10 cm
(d) none of these
Answer
A
Question. It is given that ΔABC~ ΔDEF and BC/EF=1/5 . Then (ar ΔDEF ) /( arΔABC ) is equal to
(a) 5
(b) 25
(c) 25 1
(d) 5 1
Answer
B
Question. If ΔABC~ΔDEF then which of the following is not true?
(a) BC.DF = AC.EF
(b) AB.DF = AC.DE
(c) BC.DE = AB.EF
(d) BC.DE = AB.FD
Answer
D
Question. In the given figure and ° BD AD EC AE = +ADE = 70 and +ACB = 50° then +BAC is equal to A B C D E
(a)70°
(b) 50°
(c) 80°
(d) 60°
Answer
D
Question. In the given figure AD = 3 cm, AE = 5 cm, BD = 4 cm. CE = 4 cm, CF = 2 cm, BF = 2.5 cm then which of the following is true?

(a) DE ll BC
(b) DF ll AC
(c) EF ll AB
(d) none of them
Answer
C
Question. Two triangles BAC and BDC, right angled at A and D respectively, are drawn on the same base BC and on the same side of BC. If AC and DB intersect at P, then AP × PC is equal to
(a) AB × CD
(b) AB × BC
(c) BC × CD
(d) DP × PB
Answer
D
Question. From point X, Alok walks 112 m east to reach at point Y. From point Y, Alok walks 15 m toward north to reach point Z. What is the straight-line distance between position when he started and his position now?

(a) 113 m
(b) 117 m
(c) 123 m
(d) 127 m
Answer
A
Question. XY is drawn parallel to the base BC of a ΔABC cutting AB at X and AC at Y. If AB = 4 BX and YC = 2 cm, then AY is equal to
(a) 2 cm
(b) 6 cm
(c) 8 cm
(d) 4 cm
Answer
B
Question. If in triangles ABC and DEF, AB/EF= AC/DE , then they will be similar when
(a) ∠A = ∠D
(b) ∠A = ∠E
(c) ∠B = ∠E
(d) ∠C = ∠F
Answer
B

We hope you liked the above Triangles MCQ Class 10 Mathematics. In case you have any questions please put them in the comments box below and our teachers will provide you a response.


