Determinants MCQ Questions Class 12 Mathematics
Please refer to MCQ Questions for Class 12 Mathematics Chapter 4 Determinants with answers below. These multiple-choice questions have been prepared based on the latest NCERT book for Class 12 Mathematics. Students should refer to MCQ Questions for Class 12 Mathematics with Answers to score more marks in Grade 12 Mathematics exams. Students should read the chapter Determinants and then attempt the following objective questions.
MCQ Questions for Class 12 Mathematics Chapter 4 Determinants with answers
MCQ Questions for Class 12 Mathematics Chapter 4 provided below covers all important topics given in this chapter. These MCQs will help you to properly prepare for exams.
Question: If a,b and c are in AP, then the value of

(a) x-(a+ b+ c)
(b) 9x2+a+b+c
(c) a+ b+ c
(d) 0
Answer
D
Question:

(a) 2007
(b) 2008
(c) (2008)2
(d) (2007)2
Answer
C
Question:

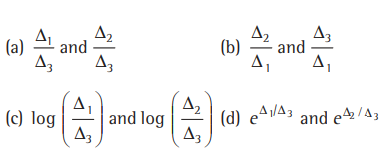
Answer
D
Question:

(a) i
(b) – ω2
(c) ω
(d) – i
Answer
A
Question: For positive numbers x y , and z, the numerical value

(a) 0
(b) 1
(c) loge xyz
(d) None of these
Answer
A
Question:

(a) AP
(b) HP
(c) GP
(d) None of these
Answer
A
Question:

(a) 1
(b) – 4
(c) 4
(d) 2
Answer
B
Question: If a, b and c are respectively the pth, qth and rth terms of an AP, then

(a) 1
(b) – 1
(c) 0
(d) pqr
Answer
C
Question: If n= 3k and 1,ω,ω2 are the cube roots of unity, then

(a) 0
(b) 1
(c) – 1
(d) None of these
Answer
A
Question: The coefficient of x in f (x)

-1<x≤1,is
(a) 1
(b) –2
(c) –1
(d) 0
Answer
B
Question: Let [x] represent the greatest integer less than or equal to x, then the value of the determinant

(a) – 8
(b) 8
(c) 10
(d) None of these
Answer
A
Question: In a Δ ABC, if

then sin2A+sin2B+sin2 C is equal to
(a) 9/4
(b) 4/9
(c) 1
(d) 3√ 3
Answer
A
Question:

(a) 5
(b)25
(c) 125
(d) 0
Answer
B
Question:

Answer
C
Question: If c<1 and the system of equations x +y-1=0,2x-y-c=0 and -bx+3by-c=0 is consistent,then the possible real values of b are

Answer
C
Question: If x,y, z are integers in AP, lying between 1 and 9 and x 51, y41 , and z31 are three digited numbers,
a) x+ y+ z
(b) x- y +z
(c) 0
(d) None of these
Answer
C
Question: If p, q,r are real, then Δ =

(a) real
(b) imaginary
(c) 0
(d) None of these
Answer
A
Question:

(a) 0
(b) Δ≠ 0
(c) Δ = f (x, y, z)
(d) None of these
Answer
A
Question: If y= sin px and yn is the nth derivative of y, then
(a) 0
(b) dependent of x
(c) non-zero constant
(d) None of these
Answer
A
Question: The system of equations x+ 2y+3z = 4, 2x+3y+4z=5, 3x+4y+5z=6 has
(a) infinitely many solutions
(b) no solution
(c) unique solution
(d) None of these
Answer
A
Question:

Answer
D
Question: If Δ =

(a) an integer
(b) a natural number
(c) an irrational number
(d) an imaginary number
Answer
A
Question:

is always non-negative. Also, find the condition for the determinant to be zero.
(a) a+ b+ c=0 or a=b=c
(b) only a+ b+ c =0
(c) only a= b= c
(d) None of the above
Answer
A
Question:

Answer
B
Question:

then the numerical quantity k should be
(a) 1
(b) 2
(c) 3
(d) 4
Answer
B
Question: All the values of t for which the system of equations.

(a) 1 or 2
(b) 2 or 4
(c) 0 or 3
(d) None of these
Answer
C
Question: The maximum value of
(a) – 2
(b) – 6
(c) 6
(d) 2
Answer
C
Question:
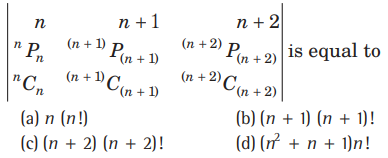
Answer
D
Question: If wis an imaginary cube root of unity, then the value

(a) a3+b3+c2-3abc
(b) a2b-b2c
(c) 0
(d) a2+b2+c2
Answer
C
Question: If the determinant

expanded in powers of sin , x then the constant term is
(a) 0
(b) 1
(c) – 1
(d) 2
Answer
C
Question: If a b, and c are cube roots of unity, then
(a) 0
(b) e
(c) e2
(d) e3
Answer
A
Question:

and the vectors(1,a,a2),(1,b,b2) and (1,c,c2) are non-coplanar, then abc is equal to
(a) 2
(b) – 1
(c) 1
(d) 0
Answer
B
Question: if A =
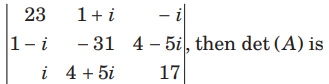
(a) complex number with positive real part
(b) complex number with negative imaginary part
(c) pure imaginary
(d) real
Answer
D
Question:

(a) x, y and z are in AP
(b) x, y and z are in GP
(c) x, y and z are in HP
(d) xy, yz and zx are in AP
Answer
B
Question: Consider the set A of all determinants of order 3 with entries 0 or 1 only. Let B be the subset of A consisting of all determinants with value 1 and C be the subset of A consisting of all determinants with value – 1.Then,
(a) C is empty
(b) B and C have the same number of elements
(c) A= B ∪ C
(d) B has twice as many elements as C
Answer
B
Question:

(a) 1
(b) – 1
(c) – 2
(d) 0
Answer
C
Question:
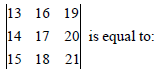
(a) 57
(b) – 39
(c) 96
(d) 0
Answer
D
Question: If A is a non-singular matrix of order 3, then |adj A| = |A|n. Here the value of n is
(a) 2
(b) 4
(c) 6
(d) 8
Answer
A
Question: If A is a square matrix such that (A – 2I) (A + I) = 0, then A–1 =
(a) A–I/2
(b) A+I/2
(c) 2 (A – I)
(d) 2A + I
Answer
A
Question: A system of linear equations
a1x + b1y = c1
a2 x + b2y = c2
can be represented in matrix form as

Answer
B
Question: If the equations x + ay – z = 0, 2x – y + az = 0, ax + y + 2z = 0 have non-trivial solutions, then a =
(a) 2
(b) – 2
(c) √3
(d) – √3
Answer
B
Question: Let A be a matrix of order 3 and let Δ denotes the value of determinant A. Then determinant (– 2A) =
(a) – 8 Δ
(b) – 2Δ
(c) 2 Δ
(d) 8 Δ
Answer
A
Question:

(a) 0
(b) –1
(c) 2
(d) 1
Answer
D
Question:

then the other factor is:
(a) (x – 2) (x – 7)
(b) (x – 2) (x – a)
(c) (x + 9) (x – a)
(d) (x + 2) (x + a)
Answer
A
ASSERTION – REASON TYPE QUESTIONS
(a) Assertion is correct, reason is correct; reason is a correct explanation for assertion:
(b) Assertion is correct, reason is correct; reason is not a correct explanation for assertion
(c) Assertion is correct, reason is incorrect
(d) Assertion is incorrect, reason is correct.
Question:

is a symmetric matrix.
Reason: For the given matrix A’ = A
Answer
A
Question:
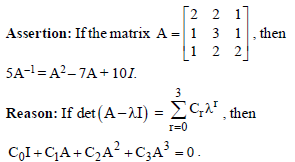
Answer
D
Question: Consider the system
x + y + z = 1
2x + 2y + 2z = 2
4x + 4y + 4z =3
Assertion: The above system has infinitely many solutions.
Reason: For the above system det A = 0 and (adj A) B = O, where

Answer
D
Question:
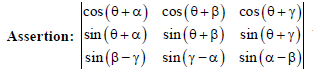
is independent of θ
Reason: If f(θ) = c, then f(θ) is independent of θ.
Answer
A
Question:

Reason: The value of determinant of matrix A is zero.
Answer
A
CASE STUDY QUESTIONS
1. Manjit wants to donate a rectangular plot of land for a school in his village. When he was asked to give dimensions of the plot, he told that if its length is decreased by 50 m and breadth is increased by 50 m, then its area will remain same, but if length is decreased by 10 m and breadth is decreased by 20 m, then its area will decrease by 5300 m2. (Image 39)
Question. The equations in terms of X and Y are
(a) x – y = 50, 2x – y = 550
(b) x – y = 50, 2x + y = 550
(c) x + y = 50, 2x + y = 550
(d) x + y = 50, 2x + y = 550
Answer
B
Question. The value of x (length of rectangular fiel(d) is
(a) 150 m
(b) 400 m
(c) 200 m
(d) 320 m
Answer
C
Question. The value of y (breadth of rectangular fiel(d) is
(a) 150 m
(b) 200 m
(c) 430 m
(d) 350 m
Answer
A
Question. How much is the area of rectangular field?
(a) 60000 sq m.
(b) 30000 sq m.
(c) 30000 m
(d) 3000 m
Answer
B
2. Raja purchases 3 pens, 2 pencils and 1 mathematics instrument box and pays ₹ 41 to the shopkeeper. His friends, Daya and Anil purchases 2 pens, 1 pencil, 2 instrument boxes and 2 pens, 2 pencils and 2 mathematical instrument boxes respectively. Daya and Anil pays ₹ 29 and ₹ 44 respectively. Based on the above information answer the following:
Question. The cost of one pen is
(a) ₹ 2
(b) ₹ 5
(c) ₹ 10
(d) ₹ 15
Answer
A
Question. The cost of one pen and one pencil is
(a) ₹ 5
(b) ₹ 10
(c) ₹ 15
(d) ₹ 17
Answer
D
Question. The cost of one pen and one mathematical instrument box is
(a) ₹ 7
(b) ₹ 10
(c) ₹ 15
(d) ₹ 18
Answer
A
Question. The cost of one pencil and one mathematical instrumental box is
(a) ₹ 5
(b) ₹ 10
(c) ₹ 15
(d) ₹ 20
Answer
D
Question. The cost of one pen, one pencil and one mathematical instrumental box is
(a) ₹ 10
(b) ₹ 15
(c) ₹ 22
(d) ₹ 25
Answer
C
3. The management committee of a residential colony decided to award some of its members (say x) for honesty, some (say y) for helping others and some others (say z) for supervising the workers to kept the colony neat and clean. The sum of all theawardees is 12. Three times the sum of awardees for cooperation and supervision added to two times the number of awardees for honesty is 33. The sum of the number of awardees for honesty and supervision is twice the number of awardeesfor helping. (Image 41)
Question. Value of x + y + z is
(a) 3
(b) 5
(c) 7
(d) 12
Answer
D
Question. Value of x − 2 y is
(a) z
(b) -z
(c) 2z
(d) -2z
Answer
B
Question. The value of z is
(a) 3
(b) 4
(c) 5
(d) 6
Answer
C
Question. The value of x + 2 y is
(a) 9
(b) 10
(c) 11
(d) 12
Answer
C
Question. The value of 2x + 3y + 5z is
(a) 40
(b) 43
(c) 50
(d) 53
Answer
B
4. Read the following text and answer the following questions on the basis of the same:
Two schools Oxford and Navdeep want to award their selected students on the values of sincerity, truthfulness and helpfulness. Oxford wants to award Ex each, y each and z each for the three respective values to 3,2 and 1 students respectively with a total award money of 1600. Navdeep wants to spend 2300 to award its 4, 1 and 3 students on the respective values (by giving the same amount to the three values as before). The total amount of the award for one prize on each is ₹900. (Image 41)
Question. Value of x + y + z is
(a) 800
(b) 900
(c) 1000
(d) 1200
Answer
B
Question. Value of 4x + y + 3z is
(a) 1600
(b) 2300
(c) 900
(d) 1200
Answer
B
Question. The value of y is
(a) 200
(b) 250
(c) 300
(d) 350
Answer
C
Question. The value of 2x + 3 y = ⋯ … … … ..
(a) 1000
(b) 1100
(c) 1200
(d) 1300
Answer
D
Question. The value of y − x = ⋯ … … … …
(a) 100
(b) 200
(c) 300
(d) 400
Answer
D
